Derivada de Arctan x

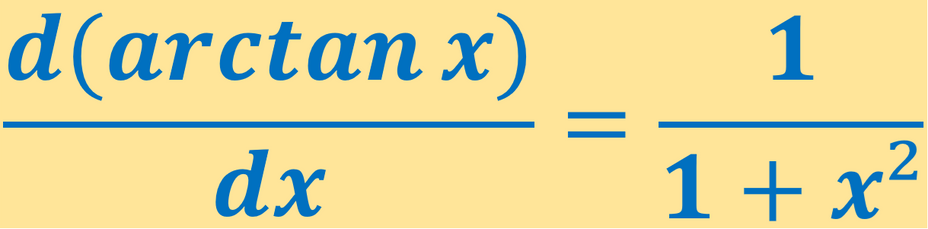
¿ Cuál es la Derivada de Arctan x ?

La derivada de arctan x es 1/1+x².

$(arctanx{)}^{\prime }=\frac{1}{1+x^2}$

$\frac{d}{dx}(arctanx)=\frac{1}{1+x^2}$

Prueba de la Derivada de Arctan x

Método 1

$f^{\prime }(x)=\underset{h\to 0}{\lim }\frac{f(x+h)-f(x)}{h}$

$(arctanx{)}^{\prime }=\underset{h\to 0}{\lim }\frac{arctan(x+h)-arctanx}{h}$

$arctan(x+h)=U$, $tanU=x+h$

$arctanx=V$, $tanV=x$

$tanU-tanV=\cancel{x}+h-\cancel{x}$

$tanU-tanV=h$

$h\to 0$, $tanU-tanV\to 0$, $tanU\to tanV$, $U\to V$

$(arctanx{)}^{\prime }=\underset{U\to V}{\lim }\frac{U-V}{tanU-tanV}$

$\boxed{tanp-tanq=\frac{sin(p-q)}{cosp.cosq}}$

$(arctanx{)}^{\prime }=\underset{U\to V}{\lim }\frac{U-V}{\frac{sin(U-V)}{cosU.cosV}}$

$(arctanx{)}^{\prime }=\underset{U\to V}{\lim }[\frac{(U-V).cosU.cosV}{sin(U-V)}]$

$(arctanx{)}^{\prime }=\underset{U\to V}{\lim }[\frac{U-V}{sin(U-V)}.cosU.cosV]$

$(arctanx{)}^{\prime }=\underset{U\to V}{\lim }\frac{U-V}{sin(U-V)}.\underset{U\to V}{\lim }cosU.\underset{U\to V}{\lim }cosV$

$U\to V$, $U-V\to 0$

$(arctanx{)}^{\prime }=\underset{U-V\to 0}{\lim }\frac{U-V}{sin(U-V)}.\underset{U\to V}{\lim }cosU.\underset{U\to V}{\lim }cosV$

$\boxed{\underset{t\to 0}{\lim }\frac{t}{sint}=1}$

$(arctanx{)}^{\prime }=1.cosV.cosV$

$(arctanx{)}^{\prime }=co{s}^{2}V$

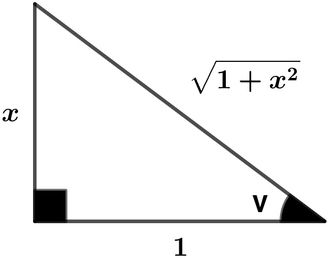
$tanV=x$

$cosV=\frac{1}{\sqrt{1+x^2}}$

$(arctanx{)}^{\prime }=(\frac{1}{\sqrt{1+x^2}}{)}^2$

$(arctanx{)}^{\prime }=\frac{1}{1+x^2}$

Método 2

$y=arctanx$

$tany=x$

$(tany{)}^{\prime }=(x{)}^{\prime }$

$\boxed{(tanu{)}^{\prime }=u^{\prime }.(1+ta{n}^{2}u)}$

$y^{\prime }.(1+ta{n}^{2}y)=1$

$y^{\prime }=\frac{1}{1+ta{n}^{2}y}$

$y^{\prime }=\frac{1}{1+x^2}$